This is part of a series of posts concerning Problems with the Rahmstorf (2007) paper.
Critique #3. Rahmstorf extrapolates out more than five times the measured temperature domain.
Extrapolation is risky business. Even when the fitted model accurately describes the real data over its domain, extrapolation beyond that domain can lead to very poor predictions. When the fitted model does not accurately describe the measured data (Rahmstorf's unbinned sea level rise rate vs. temperature, see figure 3, here, for example) the result can be truly bizarre. The NIST Engineering Handbook states:
Modeling and prediction allows us to go beyond the data to gain additional insights, but they must be done with great caution. Interpolation is generally safer than extrapolation, but mis-prediction, error, and misinterpretation are liable to occur in either case...The best attitude, and especially for extrapolation, is that the derived conclusions must be viewed with extra caution. !
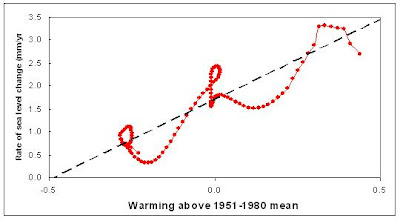
Rahmstorf's projection for future sea level (figure 4 in his paper), is reproduced in part in figure 1, below, and makes it look as if his measurement domain is 120 years and that he has extrapolated out another 100 years. But in reality, his measurement domain was in decrees C of temperature anomaly, and his range was in sea level rise rate. Extrapolating out 100 years based on 120 years of data would be bad enough, but he actually extrapolates out more that 5
°C based on 0.8 °C of data. See figure 2! This is an extrapolation of poorly fit data to over six time the measured data domain!!!
Figure 1. Reproduction of Rahmstorf's figure 4, showing "sea-level projections
from 1990 to 2100." This image gives the impression that it shows an extrapolation from measured sea level data spanning 120 years out for an additional 100 years.
Figure 2. But the real extrapolation is from the sea level rise vs temperature plot. First he fits a straight line to a twisted piece of spaghetti, then extends that line way, way out.
This type extreme form of extrapolation is best summed up by Mark Twain in Life on the Mississippi (1883). Twain writes about effect of cutting across "horseshoe curves" in the river over the years in order to shorten it.
" The Mississippi between Cairo and New Orleans was twelve hundred and fifteen miles long one hundred and seventy-six years ago. It was eleven hundred and eighty after the cut-off of 1722. It was one thousand and forty after the American Bend cut-off. It has lost sixty-seven miles since. Consequently its length is only nine hundred and seventy-three miles at present.
Now, if I wanted to be one of those ponderous scientific people, and `let on' to prove what had occurred in the remote past by what had occurred in a given time in the recent past, or what will occur in the far future by what has occurred in late years, what an opportunity is here! Geology never had such a chance, nor such exact data to argue from! Nor `development of species', either! Glacial epochs are great things, but they are vague--vague. Please observe. In the space of one hundred and seventy-six years the Lower Mississippi has shortened itself two hundred and forty two miles. This is an average of a trifle over one mile and a third per year. Therefore, any calm person, who is not blind or idiotic, can see that in the Old Oolitic Silurian Period, just a million years ago next November, the Lower Mississippi River was upward of one million three hundred thousand miles long, and stuck out over the Gulf of Mexico like a fishing-rod. And by the same token any person can see that seven hundred and forty-two years from now the Lower Mississippi will be only a mile and three-quarters long, and Cairo and New Orleans will have joined their streets together, and be plodding comfortably along under a single mayor and a mutual board of aldermen. There is something fascinating about science. One gets such wholesale returns of conjecture out of such a trifling investment of fact."
Back to series of posts concerning Problems with the Rahmstorf (2007) paper.
1. Rahmstorf, A Semi-Empirical Approach to Projecting Sea Level Rise, Science 315, 368 (2007)
Back to series of posts concerning Problems with the Rahmstorf (2007) paper.